| Es gibt z.Z. Probleme mit einem Update, es ist nur Lesen möglich. Currently there are issues with the installation, readonly possible for now. |
Difference between revisions of "Press cut"
(Filename) |
m |
||
| Line 46: | Line 46: | ||
In many of the large Educa puzzles you will find a technique to get exactly the right number of pieces. Each segment of 999 pieces (27 by 37 pieces) contains a slitted form devided into two separate smaller pieces: | In many of the large Educa puzzles you will find a technique to get exactly the right number of pieces. Each segment of 999 pieces (27 by 37 pieces) contains a slitted form devided into two separate smaller pieces: | ||
[[File:Educa 2Pieces.jpg|none|Double piece by Educa]] | [[File:Educa 2Pieces.jpg|none|Double piece by Educa]] | ||
| − | Here an example from the puzzle [[24000 Life, The greatest puzzle]]: | + | Here an example from the puzzle [[24000 Life, The greatest puzzle (1)]]: |
[[File:Educa 2Pieces1.jpg|none|Double piece of "Life"]] | [[File:Educa 2Pieces1.jpg|none|Double piece of "Life"]] | ||
| Line 58: | Line 58: | ||
====Punch repeat Educa 24.000 pieces==== | ====Punch repeat Educa 24.000 pieces==== | ||
| − | In the [[24000 Life, The greatest puzzle]] there are 4 bags of each 6.000 pieces. Each bag contains 6 punch repeats, each with 27 x 37 = 999 pieces and a double piece. | + | In the [[24000 Life, The greatest puzzle (1)]] there are 4 bags of each 6.000 pieces. Each bag contains 6 punch repeats, each with 27 x 37 = 999 pieces and a double piece. |
[[Category:Puzzle information]] | [[Category:Puzzle information]] | ||
Revision as of 12:59, 8 August 2019
| Language: | English • Deutsch |
|---|
Contents
General information
The cutting of a jigsaw is called punching. The first puzzles made of plywood were cut with a jigsaw. In the mass production one uses stencils from knives, which punch the puzzles. Other manufacturing methods use laser or waterjet techniques.
A demonstrative video about the production of a punching sheet has been published by the "Sendung mit der Maus".
Kind of press cuts
There are different types of cut patterns, the most common form of cutting is grid or raster punching.
Grid punching
In the case of a grid punching, the individual pieces lie as in a checkerboard pattern in which the edges of the individual panels have been provided with notches or bulges. Each edge of a piece touches only one edge of another piece.
This is the most common form of punching from manufacturers such as Ravensburger, Schmidt or Clementoni.
The size of the individual pieces nevertheless varies, since the alignment on a uniform grid is not exactly given. You can also determine partially how the position of a single piece is located: horizontal or vertical.
A determination of the total number of pieces of a puzzle is possible by counting the edge pieces horizontally and vertically and multiplying both.
Strip punching
In contrast to a grid punching, the individual pieces of a strip punching are not exactly opposite each other, so that more than one piece is connected to one side of a piece. The rows or columns of pieces are in stripes.
These strips can be both horizontal and vertical.
This punch was common in older puzzles of MB.
The total number of edge pieces can be determined as in the case of a grid punching.
Victorian cut
The Victorian cut came up about 1900 (therefore victorian) and in contrast to the two previous types the pieces it do not follow a grid. The piece shapes can also be very different: edge pieces without straight edges, middle pieces with straight edges or pieces in special shapes (Whimsy).
This cut is mostly used in handmade wooden puzzles, e.g. by the company Wentworth.
A determination of the total number of puzzle pieces is not possible by counting the edge pieces.
Punch repeat
For larger puzzles, it is easier to use the same punching template several times for reasons of cost and time, so that almost all pieces (except possibly the edge pieces) are available twice or more in identical shapes.
Punch repeat Educa
In many of the large Educa puzzles you will find a technique to get exactly the right number of pieces. Each segment of 999 pieces (27 by 37 pieces) contains a slitted form devided into two separate smaller pieces:
Here an example from the puzzle 24000 Life, The greatest puzzle (1):
The neighboring pieces are slightly enlarged, the rest have normal sizes.
In this actual grid punching, an exact number of 1000 parts is achieved instead of 999.
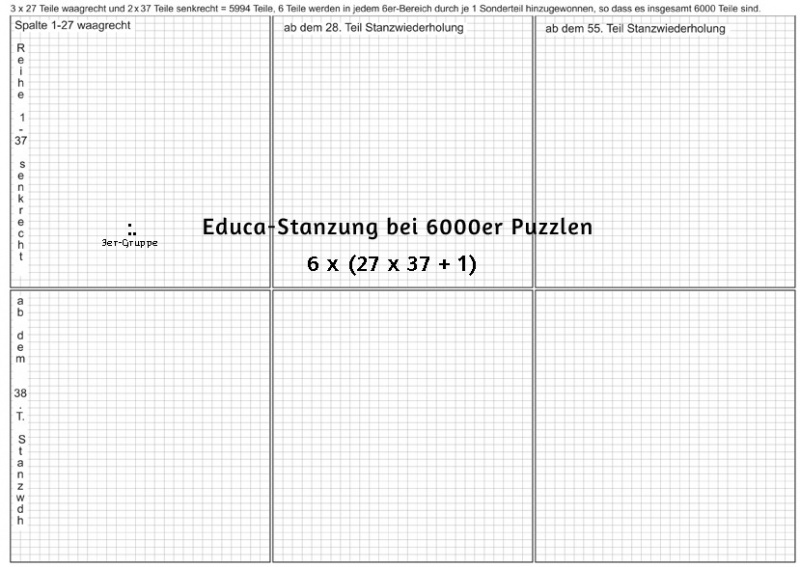
Punch repeat Educa 6.000 pieces
Punching: 6-times of 27 multiplied by 37 pieces = 999 pieces (+ respectively one double piece)
Punch repeat Educa 24.000 pieces
In the 24000 Life, The greatest puzzle (1) there are 4 bags of each 6.000 pieces. Each bag contains 6 punch repeats, each with 27 x 37 = 999 pieces and a double piece.